Question

All manufacturing processes have some variation. For example, when manufacturing batteries for a smart phone, not all batteries will have the exact same usage-hours upon leaving the factory. In other words, one battery might last 8 hours under normal conditions before needing to be charged; while another battery may last 8.2 hours (under the same conditions) before needing to be charged.

The company advertises that the life of a battery is 8 hours. To maintain quality control, they test battery life of a random sample of batteries coming out of production. The sample had a mean usage time of 8 hours and 10 minutes with a standard deviation of 25 minutes.

The manufacturer feels as though they are replacing too many batteries, and wishes to improve their manufacturing process.They wantto adjust the production process so that only 1 in 100 has a charge life that lasts less than the advertised time. What should the mean time of the manufacture batteries be in order to meet this goal(assuming the standard deviation is unchanged)?

Answer 1

The mean time of the manufacture batteries should be 432 minutes, which is 7 hours and 12 minutes = 7.2 hours.

Explanation:

Problems of normally distributed samples are solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

In this problem, we have that:

I am going to use both the mean and the standard deviation in minutes(1 hour is 60 minutes). So

`\mu = 60*8 + 10 = 490, \sigma = 25`

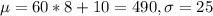
They want to adjust the production process so that only 1 in 100 has a charge life that lasts less than the advertised time. What should the mean time of the manufacture batteries be in order to meet this goal(assuming the standard deviation is unchanged)?

The new mean should be the 1st percentile, which is the value of X when Z has a pvalue of 0.01. So it is X when Z = -2.327.

`Z = (X - \mu)/(\sigma)`

`-2.327 = (X - 490)/(25)`

`X - 490 = -2.327*25`

`X = 432`

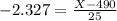
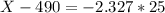
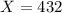
The mean time of the manufacture batteries should be 432 minutes, which is 7 hours and 12 minutes = 7.2 hours.