Given:
Let the random variable x is normally distributed with mean
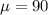 and
and
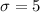
We need to determine the probability of

Probability of
 :
:
The formula to determine the value of
 is given by
is given by
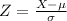
Thus, we have;
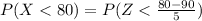
Simplifying, we get;
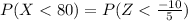
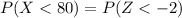
Using the normal distribution table, the value of -2 is given by 0.0228
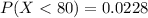
Thus, the value of
 is 0.0228
is 0.0228