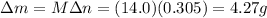Question

a balloon contains 0.950 of nitrogen gas at a volume of 25.5 L. How many grams of N2 should be released from the balloon to bring the volume to 17.3 L? ____g N2

Answer 1

4.27 g

Step-by-step explanation:

We can write that for a gas kept at constant pressure and temperature, the number of moles of the gas is directly proportional to its volume:

`n\propto V`

Which can be rewritten as

`(n_1)/(V_1)=(n_2)/(V_2)`

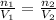
where here we have:

`n_1=0.950 mol` is the initial number of moles

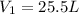`V_1=25.5 L` is the initial volume of the gas

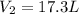`V_2=17.3 L` is the final volume of the gas

So the final number of moles is

`n_2=(n_1 V_2)/(V_1)=((0.950)(17.3))/(25.5)=0.645 mol`

So the number of moles that should be released from the balloon is:

`\Delta n = n_1-n_2=0.950-0.645=0.305 mol`

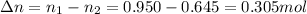
The molar mass of gas N2 is

`M=14 g/mol`

Therefore, the mass of gas that should be released is:

`\Delta m = M\Delta n =(14.0)(0.305)=4.27 g`