Answer:
His graph is incorrect, because, Jeffrey drew it with vertex (4 , -4) and x-intercepts (2 , 0) and (5 , 0), but the vertex is (3 , -4) and x-intercepts are (1 , 0) , (5 , 0)
Explanation:
The quadratic function y = ax² + bx + c is represented graphically by a parabola
- The parabola has a vertex point, the point which divides the parabola into two congruent parts
- The parabola is opened downward if the leading coefficient a is negative, then its vertex is maximum
- The coordinates of the vertex point are (h , k), where h =
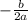 and k is the value of y at x = h
and k is the value of y at x = h - The x-intercepts are the values of x at y = 0
∵ The quadratic is f(x) = x² - 6x + 5
∴ a = 1 , b = -6 and c = 5
∵ The leading coefficient a is positive
∴ The graph of f(x) is a parabola oped upward
∴ The parabola has a minimum vertex
The vertx point of the parabola is (h , k)
∵ h =
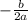
∴ h =

∴ h = 3
- To find k substitute x by 3 in f(x)
∵ f(3) = (3)² - 6(3) + 5 = 9 - 18 + 5
∴ f(3) = -4
∵ k = f(3)
∴ k = -4
That means the coordinates of the vertex of the parabola are (3 , -4)
The x-intercepts means values of x at f(x) = 0
∵ x² - 6x + 5 = 0
- Factorize it into two binomial factors
∵ x² = (x)(x)
∵ 5 = (-1)(-5)
∵ (x)(-1) + (x)(-5) = -x + -5x = -6x
- That means the factors are (x - 1) and (x - 5)
∴ The factors of x² - 6x + 5 are (x - 1) and (x - 5)
∴ (x - 1)(x - 5) = 0
Equate each factor by 0 to find the value of x
∵ x - 1 = 0
- add 1 to both sides
∴ x = 1
∵ x - 5 = 0
- add 5 to both sides
∴ x = 5
∴ The x-intercepts are (1 , 0) and (5 , 0)
That means the parabola intersects the x-axis ate points (1 , 0) and (5 , 0)
Jeffrey drew the graph with vertex (4 , -4) and x-intercepts (2 , 0) and (5 , 0), then his graph is incorrect