Answer:
The three pieces of wood when placed together cannot form a triangle when used for full length.
Explanation:
Given:
Length of Board A = 5 inches
Length of Board B = 7 inches
Length of Board C = 13 inches
We need to find whether three pieces of wood be placed together to form a triangle.
Solution:
Now we know that;
By Triangle inequality property:
"A triangle can be form when sum of the length of the 2 side of triangle is greater than third."
For this all three condition must be fulfilled.
We will check for each condition whether it is true or not.
Condition 1: Board A + Board B > Board C
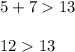
Which is false.
Condition 2: Board B + Board C > Board A
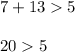
Which is true.
Condition 3: Board C + Board A > Board B
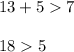
Which is true.
From above we can see that 2 conditions are true and 1 is false and for triangle creation all 3 conditions must be true.
Hence The three pieces of wood cannot be placed together to form a triangle when used for full length.