Answer:
Area of square pyramid is
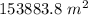 .
.
Explanation:
Diagram of the given scenario is shown below,
Given that,
The Great Pyramid in Giza, Egypt is a square pyramid. The area of base shape is made up with square is
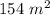 . The side of the square is
. The side of the square is
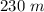 and The height of each triangle is
and The height of each triangle is
 .
.
So, Area of Square shape =
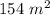
Side of square =
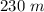
Height of each triangle =

Finding the Height of triangular shape which is here known as slant height.
In Δ ABO, applying Right angle pythagorean Theorem,
Base
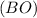 =
=

Height
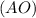 =
=
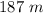
Now,
∴
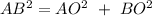
⇒
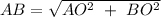
⇒
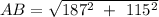
⇒
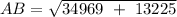
⇒
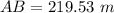
Then,
Area of square pyramid =
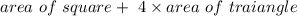
=
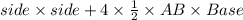
=
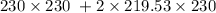
=
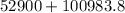
=

Hence, Area of square pyramid is
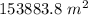 .
.