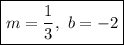Question

Match the correct y=mx+b equation to the graph:

pls show work/explanation!

Answer 1

`\displaystyle y=(1)/(3)x-2`

`\displaystyle m=(1)/(3),\ b=-2`

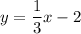
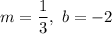
Explanation:

Equation of a line

We only need two points through which it passes to define the equation of a line. The graph provided in the question shows two clear points to pick: (0,-2) and (3,-1)

Now we wet up the equation of the line by using the formula

`\displaystyle y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)`

Plugging in the points:

`\displaystyle y-(-2)=(-1-(-2))/(3-0)(x-0)`

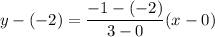
Operating

`\displaystyle y+2=(-1+2)/(3)(x)`

`\displaystyle y=(1)/(3)x-2`

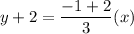
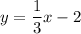
The values of m and b are

`\displaystyle \boxed{m=(1)/(3),\ b=-2}`