Answer:
Area of pentagon
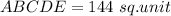 .
.
Explanation:
Labelled Diagram of given shape is shown below,
Given that,
A regular pentagon is divided into congruent triangle. each triangle has an area of
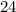
 .
.
So, Regular pentagon has an all sides are equal and all the corresponding angles are also equal. pentagon has an each angle measure is
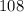 ° and side says
° and side says
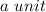 .
.
Here, According to Question
All triangles are congruent then there area will be same.
∴ area of pentagon
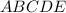 = area of Δ
= area of Δ
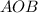 + area of Δ
+ area of Δ
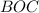 + area of
+ area of
Δ
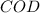 + area of Δ
+ area of Δ
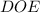 + area of Δ
+ area of Δ
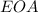 .
.
=
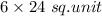 {Given that}
{Given that}
=
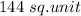
Hence,
Area of pentagon
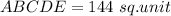 .
.