Question

A conveyor belt carries bales of hay from the ground to the loft of a barn 27 feet

above ground, as shown below. If the belt makes a 30° angle with the ground,
how far does the bale of hay travel on the conveyor belt?

Answer 1

Given:

A conveyor belt carries bales of hay from the ground to the loft of a barn 27 feet above ground.

The belt makes an 30° angle with the ground.

We need to determine the distance that the bale of hay travel on the conveyor belt.

Distance that the bale of hay travel on the conveyor belt:

The distance that the bale of hay travel on the conveyor belt can be determined using the trigonometric ratio.

Let the distance that the bale of hay travel on the conveyor belt be x.

Thus, we have;

`sin \ \theta=(opp)/(hyp)`

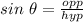
Substituting
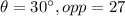`\theta=30^(\circ), opp=27` and
`hyp=x`

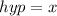
Thus, we get;

`sin \ 30^(\circ)=(27)/(x)`

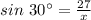
Simplifying, we get;

`x=(27)/(sin \ 30^(\circ))`

`x=(27)/(((1)/(2)))`

`x=54`

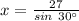
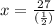
Thus, the value of x is 54 feet.

Hence, the distance that the bale of hay travel on the conveyor belt is 54 feet.