Question

If b varies jointly with a and c and b = 112 when a = 12 and c = 7, find a when b = 72 and c = 2

Answer 1

The calculated value of a in the joint variation is 27

How to determine the value of a in the variation

From the question, we have the following parameters that can be used in our computation:

b varies jointly with a and c

This means that

b = kac

Where

k is the constant of variation

b = 112 when a = 12 and c = 7

So, we have

112 = k * 12 * 7

112 = k * 84

k = 112/84

k = 4/3

Recall that

b = kac

So, we have

b = 4ac/3

Make a the subject

a = 3b/4c

When b = 72 and c = 2, we have

a = (3 * 72)/(4 * 2)

Evaluate

a = 27

Hence, the value of a is 27

Answer 2

Given:

b jointly varies with a and c. This can be written as,

`b=kac`

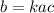
where k is a constant.

We need to determine the value of a when b = 72 and c = 2

Value of k:

Also, given that the value b = 112 when a = 12 and c = 7.

Hence, substituting these values in the expression
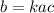`b=kac`, we get;

`112=k(12)(7)`

`112=84k`

`(4)/(3)=k`

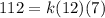
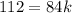
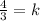
Thus, the value of k is
`k=(4)/(3)`

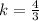
Value of a:

The value of a can be determined by substituting b = 72, c = 2 and
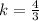`k=(4)/(3)` in the expression
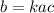`b=kac`, we have;

`72=(4)/(3)a(2)`

`72=(8)/(3)a`

`72* (3)/(8)=a`

`9=a`

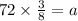
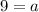
Therefore, the value of a is 9.