The stone reaches a height of 13 m when
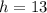 :
:

The question refers to the moment at which it **rises** to 13 m, so we need to take the earlier instance,
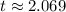 seconds after the stone is tossed.
seconds after the stone is tossed.
The stone's velocity is given by the derivative of its height function:
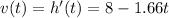
Plug in the time found above; the stone's velocity at this moment is about 4.57 m/s.