Answer : The limiting reagent in this reaction is,
 and number of moles of excess reagent is, 1.69 moles
and number of moles of excess reagent is, 1.69 moles
Explanation : Given,
Mass of
 = 500.0 g
= 500.0 g
Mass of
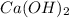 = 450.0 g
= 450.0 g
Molar mass of
 = 342.15 g/mol
= 342.15 g/mol
Molar mass of
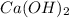 = 74.1 g/mol
= 74.1 g/mol
First we have to calculate the moles of
 and
and
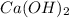 .
.
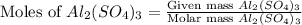
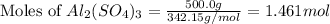
and,
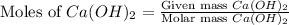
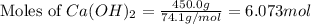
Now we have to calculate the limiting and excess reagent.
The given chemical reaction is:

From the balanced reaction we conclude that
As, 1 mole of
 react with 3 mole of
react with 3 mole of
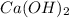
So, 1.461 moles of
 react with
react with
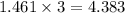 moles of
moles of
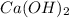
From this we conclude that,
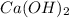 is an excess reagent because the given moles are greater than the required moles and
is an excess reagent because the given moles are greater than the required moles and
 is a limiting reagent and it limits the formation of product.
is a limiting reagent and it limits the formation of product.
Number of moles of excess reagent = 6.073 - 4.383 = 1.69 moles
Therefore, the limiting reagent in this reaction is,
 and number of moles of excess reagent is, 1.69 moles
and number of moles of excess reagent is, 1.69 moles