Answer:
It will take 0.8333h for the mustang to catch up to the grand am.
The two cars will have traveled 66.664 miles on the turnpike
Explanation:
The position for each car can be modeled by equations in the following format:
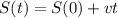
In which S(t) is the position t hours after the last car(the Ford Mustang) has left, S(0) is the position of each car when the Ford Mustang left and v is the speed of each car, in miles per hour.
Pontiac
Traveling at 50 mph, which means that
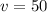 .
.
The Ford Mustang leaves half an hour after the Pontiac. The Pontiac travels at 50 mph, which means that in this interval, the Pontiac will have traveled 0.5*50 = 25 mph. This means that S(0) = 25. So
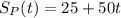
Ford Mustang
Leaves from position 0, so S(0) = 0.
Travel in the same distance at 80 mph, so
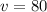 . So
. So
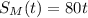
How long would it take for the mustang to catch up to the grand am ?
This is t when

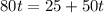
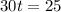
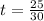
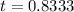
It will take 0.8333h for the mustang to catch up to the grand am.
When it catch up how far will the two cars have traveled on the turnpike
This is either
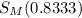 or
or
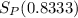 , since they will be in the same position. So
, since they will be in the same position. So
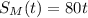

The two cars will have traveled 66.664 miles on the turnpike