Answer:
The dimensions of the box are 5.67 cm by 5.67 cm by 8.51 cm.
The total minimum cost = 28.97 cents.
Explanation:
Let the base dimensions are a cm by a cm and the height is h cm.
So, a²h = 274 ............. (1)
And, total cost, C = 0.3a² + 0.1 × 4ah = 0.3a² + 0.4ah
C = 0.3a² + 0.4 × (274/a) ................. (2)
Now, for minimum total cost, the condition is
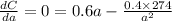
⇒
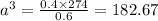
⇒ a = 5.67 cm
So,
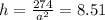 cm.
cm.
Therefore, the dimensions of the box are 5.67 cm by 5.67 cm by 8.51 cm.
And the total minimum cost =
 cents. (Answer)
cents. (Answer)