Question

An electron travels north at a velocity of 3.0 × 107 m⁄s into a magnetic field of 0.070 T pointing exactly southeast. An electron has a negative charge of 1.6 × 10–19 C. What is the magnitude of the force on the electron and the direction of this force when the electron enters the field?

A.
3.4 × 10–13 N; upward
B.
3.4 × 10–13 N; downward
C.
2.4 × 10–13 N; upward
D.
2.4 × 10–13 N; downward
E.
0 N

Answer 1

C. 2.4 × 10–13 N; upward

Step-by-step explanation:

When a charged particle moves in a region with a magnetic field, the particle experiences a force whose magnitude is given by

`F=qvB sin \theta`

where

q is the charge of the particle

v is its velocity

B is the strength of the magnetic field

`\theta` is the angle between the directions of v and B

In this problem:

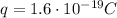`q=1.6\cdot 10^(-19)C` is the charge of the electron

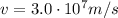`v=3.0\cdot 10^7 m/s` is its speed

`B=0.070 T` is the strength of the magnetic field

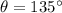`\theta=135^(\circ)` is the angle between the velocity and the field (the field is southeast, the velocity is north)

So, the magnitude of the force is:

`F=(1.6\cdot 10^(-19))(3.0\cdot 10^7)(0.070)(sin 135^(\circ))= 2.4\cdot 10^(-13) N`

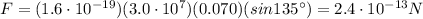
The direction of the force is perpendicular to both the motion of the particle and the component of the field perpendicular to the motion.

To find the direction, we need to use the right-hand rule. We have:

- Index finger: direction of motion --> north

- Middle finger: direction of the component of the field perpendicular to the motion --> east

- Thumb: direction of the force --> downward

However, the charge of the electron is negative, so we have to reverse the direction of the force: therefore, the force on the electron is upward.