Given:
ΔABC
 ΔDEF
ΔDEF
To find:
The length of median CP
Solution:
In ΔABC,
AP = 12, BP = 12 and PC = 3x - 12
In ΔDEF,
DQ = 16, QE = 16 and FQ = 2x + 8
If two triangles are similar, then their median is proportional to the corresponding sides.
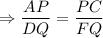
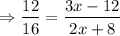
Do cross multiplication.
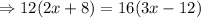

Add 192 on both sides.
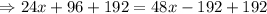
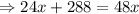
Subtract 24x from both sides.
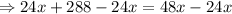
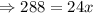
Divide by 24 on both sides.
⇒ 12 = x
Substitute x = 12 in CP.
CP = 3(12) - 12
= 36 - 12
= 24
The length of median CP is 24.