Question

A recent study reported that 18- to 24-year-olds average 192 restaurant visits per year. Assume that the standard deviation for number of visits per year for this age group is 56.5. To validate these findings, a random sample of forty 18- to 24-year-olds was selected and found to average 212 restaurant visits per year. Which of the following statements is correct

A.)The interval that contains 95% of the sample means is 170.3 and 213.7 visits. Because the sample mean is between these two values, we have support for the results of the May 2011 study.

B.)The interval that contains 95% of the sample means is 170.3 and 213.7 visits. Because the sample mean is between these two values, we do not have support for the results of the May 2011 study.

C.)The interval that contains 95% of the sample means is 174.5 and 209.5 visits. Because the sample mean is not between these two values, we have support for the results of the May 2011 study.

D.)The interval that contains 95% of the sample means is 174.5 and 209.5 visits. Because the sample mean is not between these two values, we do not have support for the results of the May 2011 study.

Answer 1

Option C) is the correct answer.

Explanation:

We are given the following in the question:

Mean = 192

Sample mean,
`\bar{x}` = 212

Sample size, n = 40

Alpha, α = 0.05

Population standard deviation, σ = 56.5

95% Confidence interval:

`\mu \pm z_(critical)(\sigma)/(√(n))`

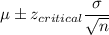
Putting the values, we get,

`z_(critical)\text{ at}~\alpha_(0.05) = 1.96`

`192 \pm 1.96((56.5)/(√(40)) ) = 192 \pm 17.5 = (174.5,209.5)`

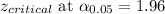
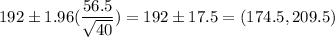
Thus, the correction answer is

Option C)

"The interval that contains 95% of the sample means is 174.5 and 209.5 visits. Because the sample mean is not between these two values, we have support for the results of the May 2011 study."