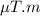Question

A long straight wire carrying a 2.5 A current passes through the centre of a rectangle of dimensions 2.5 m by 7.5 m at the angle of 45 degrees to the surface normal. What is the path integral of ∮B⇀ ∙ ds⇀ around the outside of the rectangle? Express your answer using two significant figures. The answer should be in μT∙m.

Answer 1

The value of path integral is 2.2
`\mu T.m`

Step-by-step explanation:

Given:

Current carrying by long wire
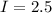`I = 2.5` A

Area of rectangle
`A = 18.75`
`m^(2)`

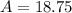
Angle with surface normal
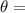`\theta =` 45°

According to the ampere's circuital law,

`\int\limits {B} \, ds = \mu _(o) I_(net)`

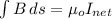
Where
`\mu _(o) = 4\pi * 10^(-7)`
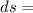`ds=` area element

Here,
`I _(net) = I \cos 45`

`I_(net) = 2.5 * (1)/(√(2) )`

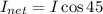
Put value of current in above equation,

`\int\limits {B} \, ds = 4\pi * 10^(-7) * 2.5 * (1)/(√(2) )`

`\int\limits {B} \, ds = 22.2 * 10^(-7)`

`\int\limits {B} \, ds = 2.2 * 10^(-6)`

`\int\limits {B} \, ds = 2.2`
`\mu T.m`

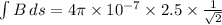
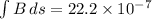
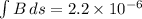
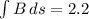
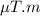
Therefore, the value of path integral is 2.2
`\mu T.m`