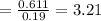Question

Now, let's finish the calculation and the determination of the formula of the iron compound: Calculate the % water of hydration : Tries 0/3 Calculate the following for Fe3 : g in 100 g sample mol in 100 g sample mol/mol Fe (3 sig figs) mol/mol Fe (whole number) Tries 0/3 Calculate the following for K : g in 100 g sample mol in 100 g sample mol/mol Fe (3 sig figs) mol/mol Fe (whole number) Tries 0/3 Calculate the following for C2O42-: g in 100 g sample mol in 100 g sample mol/mol Fe (3 sig figs) mol/mol Fe (whole number) Tries 0/3 Calculate the following for H2O g in 100 g sample mol in 100 g sample mol/mol Fe (3 sig figs) mol/mol Fe (whole number)

Answer 1

Complete Question

The complete question is shown on the first and second uploaded image

Answer:

A

The percentage of water of hydration is
`P_h= 11.01`%

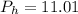Mass of
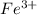`Fe^(3+)` in 100mg is 10.60mg

Moles of
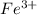`Fe^(3+)` in 100mg is
`n_i= 0.19`

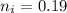
mol / mol Fe (3 sig figs) is
`= 1.00`

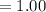
mol / mol Fe (whole number) is = 1

B

Mass of
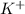`K^(+)` in 100mg is 27.70mg

Moles of
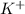`K^(+)` in 100mg is
`n_i= 0.581 moles`

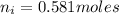
mol of K / mol of Fe (3 sig figs) is
`= 3.05`

mol of K / mol of Fe (whole number) is
`=3`

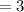
C

Mass of
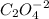`C_2O_4^(-2)` in 100mg is 55.69 mg

Moles of
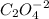`C_2O_4^(-2)` in 100mg is
`n_i= 0.633 moles`

mol of
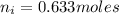
`C_2O_4^(-2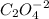)` / mol of Fe (3 sig figs) is
`= 3.33`

mol of
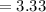
`C_2O_4^(-2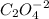)` / mol of Fe (whole number) is
`=3`

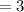
D

Mass of water in 100mg is 11.01 mg

Moles of water in 100mg is
`n_i= 0.611 moles`

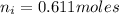
mol of water / mol of Fe (3 sig figs) is
`= 3.21`

mol of water / mol of Fe (whole number) is
`=3`

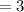
Step-by-step explanation:

The percentage of water of hydration is mathematically represented as

`P_h = 100 - (Pi + P_p + P_o)`

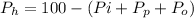
Now substituting 10.60% for
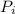`P_i` (percentage of iron ) , 22.70% for
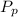`P_p`(Percentage of potassium) , 55.69% for
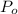`P_o` (percentage of Oxlate)

`P_h =100 - (10.60 + 22.70+55.69)`

`P_h= 11.01`%

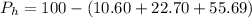
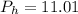For IRON

Since the percentage of
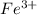`Fe^(3+)` is 10.60% then in a 100 mg of the sample the amount of
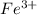`Fe^(3+)` would be 10.60 mg

Now the no of moles is mathematically denoted as

`n = (mass)/(molar \ mass )`

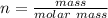
The molar mass of
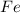`Fe` is 55.485 g/mol

So the number of moles of
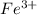`Fe^(3+)` in 100mg of he sample is

`n_i = (10.60)/(55.485)`

`n_i= 0.19`

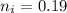
mol / mol Fe (3 sig figs) is
`= (0.19)/(0.19) = 1.00`

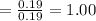
FOR POTASSIUM

Since the percentage of
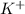`K^(+)` is 22.70% then in a 100mg of the sample the amount of
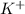`K^(+)` would be 22.70mg

The molar mass of
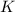`K` is 39.1 g/mol

So the number of moles of
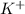`K^(+)` in 100mg of he sample is

`n_i = (22.70)/(39.1)`

`=0.581 moles`

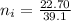
mol of K / mol of Fe (3 sig figs) is
`= (0.581)/(0.19) = 3.05`

FOR OXILATE
`C_2O_4^(-2)`

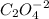
Since the percentage of
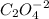`C_2O_4^(-2)` is 55.69% then in a 100mg of the sample the amount of
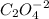`C_2O_4^(-2)` would be 55.69 mg

The molar mass of
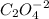`C_2O_4^(-2)` is 88.02 g/mol

So the number of moles of
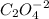`C_2O_4^(-2)` in 100mg of he sample is

`n_i = (55.69)/(88.02)`

`=0.633 moles`

mol of
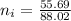
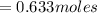
`C_2O_4^(-2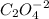)` / mol of Fe (3 sig figs) is
`= (0.633)/(0.19) = 3.33`

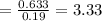
FOR WATER OF HYDRATION

Since the percentage of water is 11.01% then in a 100mg of the sample the amount of water would be 11.0 mg

The molar mass of water is 18.0 g/mol

So the number of moles of water in 100mg of he sample is

`n_i = (11.01)/(18.0)`

`=0.611 moles`

mol of water / mol of Fe (3 sig figs) is
`= (0.611)/(0.19) = 3.21`