Answer:
4.77 is the pH of the given buffer .
Step-by-step explanation:
To calculate the pH of acidic buffer, we use the equation given by Henderson Hasselbalch:
![pH=pK_a+\log(([salt])/([acid]))](https://img.qammunity.org/2021/formulas/biology/college/6usxe642bp3w274zbcv30her0kcessu95f.png)
![pH=-\log[K_a]+\log(([salt])/([acid]))](https://img.qammunity.org/2021/formulas/chemistry/college/ymoqlzvirz8m4qgz8r5dyux503q0vje8rx.png)
![pH=-\log[K_a]+\log(([CH_3CH_2COONa])/([CH_3CH_2COOH]))](https://img.qammunity.org/2021/formulas/chemistry/college/g1e6ro9avyjw8p3klktsuwxv2tme63hkuu.png)
We are given:
 = Dissociation constant of propanoic acid =
= Dissociation constant of propanoic acid =
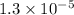
![[CH_3CH_2COONa]=0.254 M](https://img.qammunity.org/2021/formulas/chemistry/college/pv0hiqzgfdcka3d4xsm3827pgz2364x30k.png)
![[CH_3CH_2COOH]=0.329 M](https://img.qammunity.org/2021/formulas/chemistry/college/8ghsj6tal3sq3v7apbe0vsrr6m1rtxd7k5.png)
pH = ?
Putting values in above equation, we get:
![pH=-\log[1.3* 10^(-5)]+\log(([0.254 M])/([0.329]))](https://img.qammunity.org/2021/formulas/chemistry/college/kcbnm9y8mc4lj4i0u4ppjghkxi38v1mww4.png)
pH = 4.77
4.77 is the pH of the given buffer .