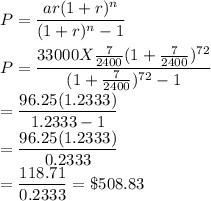Question

janelle wishes to finance a car for $33,000. the bank's annual interest rate is 3.5%, and she can choose between durations of five or six years. calculate the monthly payment and total amount paid for both duration options.use the formula,p = ar (1+r)^n/(1+r)^n-1where a is the amount to finance, r is the monthly interest rate, and n is the number of months to pay. show all of your steps.

Answer 1

For a duration of 5 years, Monthly Payment =$600.42

For a duration of 6 years, Monthly Payment =$508.83

Explanation:

`P=(ar (1+r)^n)/((1+r)^n-1) \\`

where a= Amount to Finance=$33,000

Annual interest rate = 3.5%=0.035

r=Monthly Interest Rate= 0.035 ÷ 12 =
`(7)/(2400)`

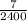
n=number of months to pay

For a duration of 5 years

n=5X12=60 months

`P=(ar (1+r)^n)/((1+r)^n-1) \\\\P=(33000 X(7)/(2400) (1+(7)/(2400) )^(60))/((1+(7)/(2400))^(60)-1) \\=(96.25 (1.1909))/(1.1909-1)\\=(96.25 (1.1909))/(0.1909)\\=(114.62)/(0.1909)=\$600.42`

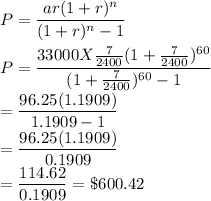
For a duration of 6 years

n=6X12=72 months

`P=(ar (1+r)^n)/((1+r)^n-1) \\\\P=(33000 X(7)/(2400) (1+(7)/(2400) )^(72))/((1+(7)/(2400))^(72)-1) \\=(96.25 (1.2333))/(1.2333-1)\\=(96.25 (1.2333))/(0.2333)\\=(118.71)/(0.2333)=\$508.83`