Answer:
19.29 m/s
Step-by-step explanation:
Apply the conservation of linear momentum in north-south direction:
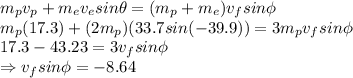
Apply the conservation of linear momentum in east-west direction:
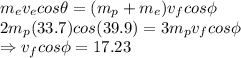
Dividing the two equations:
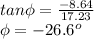
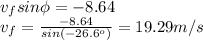
Thus, the speed of the eagle immediately after it catches its prey is 19.29 m/s.