Question

Calculate the pH for the following weak acid. A solution of HCOOH has 0.12M HCOOH at equilibrium. The Ka for HCOOH is 1.8×10−4. What is the pH of this solution at equilibrium?

Answer 1

the pH of HCOOH solution is 2.33

Step-by-step explanation:

The ionization equation for the given acid is written as:

`HCOOH\leftrightarrow H^++HCOO^-`

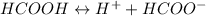
Let's say the initial concentration of the acid is c and the change in concentration x.

Then, equilibrium concentration of acid = (c-x)

and the equilibrium concentration for each of the product would be x

Equilibrium expression for the above equation would be:

`\Ka= ([H^+][HCOO^-])/([HCOOH])`

`1.8*10^-^4=(x^2)/(c-x)`

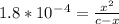
From given info, equilibrium concentration of the acid is 0.12

So, (c-x) = 0.12

hence,

`1.8*10^-^4=(x^2)/(0.12)`

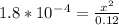
Let's solve this for x. Multiply both sides by 0.12

`2.16*10^-^5=x^2`

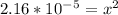
taking square root to both sides:

`x=0.00465`

Now, we have got the concentration of
`[H^+] .`

`[H^+] = 0.00465 M`

We know that,
`pH=-log[H^+]`

pH = -log(0.00465)

pH = 2.33

Hence, the pH of HCOOH solution is 2.33.

Answer 2

The correct answer is 2.34

Step-by-step explanation:

HCOOH is formic acid. It is a weak acid so it does not dissociates completely in water. At the beggining (I) the initial concentration is 0.12 M. In water it will dissociate in a certain grade x as follows:

HCOOH → H⁺ + HCOO⁻

I 0.12 M 0 0

C - x x x

E (0.12 M - x) x x

The mathematical expression for the equilibrium constant (Ka) is the following:

`K_(a) = ([H^(+) ][HCOO^(-) ])/([HCOOH])`

`1.8 x 10^(-4) = ((x x))/((0.12 M -x))`

As the value of Ka is too small in comparison with the initial concentration 0.12 M, we can approximate: 0.12 M - X ≅ 0.12 M. Then, we calculate x:

1.8 x 10⁻⁴ = x²/0.12 M

⇒ x=
`\sqrt{0.12 x 1.8 x 10^(-4) }`= 4.65 x 10⁻³

Since x = 4.65 x 10⁻³ , from the equilibrium we have:

[H⁺] = x = 4.65 x 10⁻³

From the definition of pH, we have:

pH = -log [H⁺] = -log (4.65 x 10⁻³)= 2.34