To solve this problem, apply the concepts related to the centripetal acceleration as the equivalent of gravity, and the kinematic equations of linear motion that will relate the speed, the distance traveled and the period of the body to meet the needs given in the problem. Centripetal acceleration is defined as,
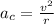
Here,
v = Tangential Velocity
r = Radius
If we rearrange the equation to get the velocity we have,

But at this case the centripetal acceleration must be equal to the gravitational at the Earth, then
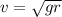

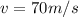
The perimeter of the cylinder would be given by,
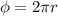
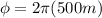

Therefore now related by kinematic equations of linear motion the speed with the distance traveled and the time we will have to
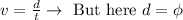
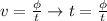
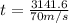
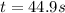
Therefore the period will be 44.9s