Answer:
11.52cm
Step-by-step explanation:
The velocity, v, of a wave undergoing a simple harmonic motion is related to the wavelength, λ, and frequency, f, of the wave as follows;
v = f x λ -----------------(i)
But;
The velocity is also given as the ratio of the length, l, of the body producing the wave to the time taken, t, to undergo the motion. i.e
v =
 --------------(ii)
--------------(ii)
Now, substitute the value of v in equation (ii) into equation (i) as follows;
 = f x λ ----------------(iii)
= f x λ ----------------(iii)
From the question,
l = 4.32m
t = 0.5s
f = 75Hz
Substitute these values into equation (iii) as follows;
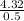 = 75 x λ
= 75 x λ
Make λ subject of the formula;
λ =
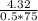
λ = 0.1152m
Convert the value to cm by multiplying by 100
λ = 0.1152 x 100cm = 11.52cm
Therefore, the wavelength of the waves on the string is 11.52cm