Answer:
Event is not unusual(p>0.05).
Explanation:
Given that :
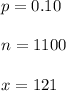
#The sample proportion is calculated as:

#Mathematically, the z-value is the value decreased by the mean then divided the standard deviation :
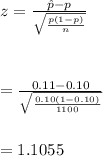
#We use the normal probability table to determine the corresponding probability;
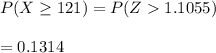
Hence, the probaility is more than 0.05, thus the event not unusual and thus this is not necessarily evidence that the proportion of Americans who are afraid to fly has increased.