Question

Please help me solve just for the answer anything helps M varies inversely as the square of P. If M is 9 when P is 2, then find M when P is 3.

Answer 1

4

Explanation:

When M varies inversely P squared rhen this is represented as

`M=\frac {k}{P^(2)}`

Here, k is a constant of proportionality

Now when M is 9 and P is 2, P squared will be 4. Now substituting the value of P squared and M into the equation we can find the xonstant of proportionality as

K=9*4=36

Now that we have k, when P is 3 then we substitute the value of P as 3 and k as 36 into the first equation hence

`M=\frac {36}{3^(2)}=4`

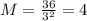
Therefore, M=4