Question

One of the parks mounds is 63 feet in height. If the top of the mound is rectangular in shape with a perimeter of 322 yards, what could be the side lengths of the rectangle?

Answer 1

see the explanation

Explanation:

we know that

The perimeter of rectangle is given by the formula

`P=2(L+W)`

where

L is the length

W is the width

In this problem we have

`P=322\ yd`

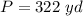
substitute

`322=2(L+W)`

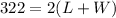
simplify

`L+W=161\ yd`

so

The side lengths of the rectangle could be any pair of values whose sum must equal 161 yards

see the attached figure to better understand the problem

With the given height approximate the length of the rectangle

`L=144\ ft`

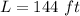
Convert to yards

`1\ yd=3\ ft`

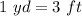
so

`144\ ft=144/3=48\ yd`

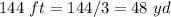
Find the width

`48+W=161\\W=161-48\\W=113\ yd`

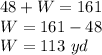
therefore

The side lengths of the rectangle could be 48 yd by 113 yd