Question

A 0.5kg stone moving north at 4 m/s collides with a 4kg lump of clay moving west at 1 m/s. The stone becomes embedded in the clay. What is the velocity (magnitude and direction) of the composite body after the collision?

Answer 1

The velocity of the composite body is 0.99m/s 63.43° west of north.

Step-by-step explanation:

Here the law of conservation of energy says that

`(1).\: \: m_1v_1cos(0)+m_2v_2cos(90^o)=(m_1+m_2)v_f cos(\theta)`

`(2).\: \: m_1v_1sin(0)+m_2v_2sin(90^o)=(m_1+m_2)v_f sin(\theta)`

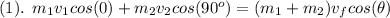
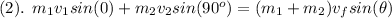
where
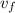`v_f` is the final velocity if the composite body, and
`\theta` is measured from west of north.

Putting in numbers and simplifying the above equation we get:

`(3).\: \: m_1v_1=(m_1+m_2)v_f cos(\theta)`

`(4).\: \: m_2v_2=(m_1+m_2)v_f sin(\theta)`

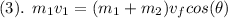
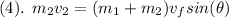
dividing equation (4) by (3) gives

`( m_2v_2=(m_1+m_2)v_f sin(\theta))/( m_1v_1=(m_1+m_2)v_f cos(\theta))`

`(m_2v_2)/(m_1v_1) = (sin(\theta))/( cos(\theta))`

`(5).\: \: tan(\theta) = (m_2v_2)/(m_1v_1)`

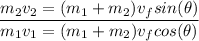
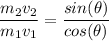
putting in
`m_1 = 0.5kg`,
`v_1 = 4m/s`,
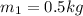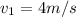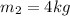`m_2 = 4kg`, and
`v_2 = 1m/s` we get:

`tan(\theta) = ((4kg)(1m/s))/((0.5kg)(4m/s))`

`\boxed{\theta = 63.43^o}`

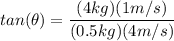
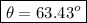
Thus, the final velocity
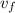`v_f` we get from equation (3) is:

`v_f=(m_1v_1)/((m_1+m_2)cos(\theta))`

`v_f=((0.5kg)(4m/s))/((4kg+0.5kg)cos(63.43^o))`

`\boxed{v_f = 0.99m/s}`

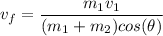
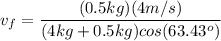
Thus, the velocity of the composite body is 0.99m/s 63.43° west of north.