Question

A bag contains 52 balls lettered from a to z and A to Z (i.e. uppercase or lowercase letters). One ball is withdrawn. What is the probability that the ball will have a letter that is lower case or earlier in the alphabet than d (or D)?

Answer 1

the probability that the ball will have a letter that is lower case or earlier in the alphabet than d (or D) is 0.557

Explanation:

Here we have ,A bag contains 52 balls lettered from a to z and A to Z (i.e. uppercase or lowercase letters). One ball is withdrawn. We need to find What is the probability that the ball will have a letter that is lower case or earlier in the alphabet than d (or D)? Let's find out:

There are 26 lowercase letters and 26 uppercase letters ! According to question we need to choose a ball which is either a lowercase ( i.e. from 26 letters ) or earlier in the alphabet than d ( or D ) which is A or B or , C .

Probability = (Favorable outcome)/(Total outcome)

Favorable outcome = 26+3=29

Total Outcome = 52

So ,

⇒
`Probability = (29)/(52)`

⇒
`Probability = 0.557`

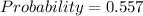
Therefore , the probability that the ball will have a letter that is lower case or earlier in the alphabet than d (or D) is 0.557