Question

a statistics professor finds that when she schedules an office hour for student help, an average of 2.4 students arrive. find the probability that in a randomly selected office hour, the number of student arrivals is 2

Answer 1

P=0.2613

Explanation:

-Notice that this is a poison probability distribution problem.

-The Poisson probability function is expressed as:

`P(X=x)=(\lambda^x e^(-\lambda))/(x!)`

where:

• x=0,1,2,3
• 
  `e` Euler's constant
• 
  `\lambda` =mean number of occurrences.

Given that x= 2 and
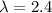`\lambda=2.4`, the probability is calculated as:

`P(X=2)=(\lambda^xe^(-\lambda))/(x!)\\\\=(2.4^2e^(-2.4))/(2!)\\\\\\=0.2613`

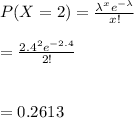
Hence, the probability that in a randomly selected office hour, the number of student arrivals is 2 is 0.2613