Question

If a projectile is fired straight upward from the ground with an initial speed of 96 feet per​ second, then its height h in feet after t seconds is given by the function ​h(t)= -16t^2 + 96t. Find the maximum height of the projectile.

Answer 1

144 feet

Explanation:

The quadratic equation is:

`h(t)=-16t^2+96t`

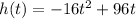
The general form of a quadratic is
`ax^2+bx+c`

So, we can match the equations and say:

a = -16

b = 96

c = 0

Now, for quadratic equations, the max value occurs at
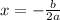`x=-(b)/(2a)` and the max value is what we get when we put that number in the function. First, lets find the value on which is occurs:

`x=-(b)/(2a)\\x=-(96)/(2(-16))\\x=3`

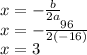
Now, put x = 3 into the equation:

`h(t)=-16t^2+96t\\h(3)=-16(3)^2+96(3)\\h(3)=144`

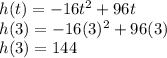
The max height of projectile is 144 feet