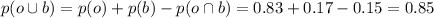Answer:
0.85
Explanation:
Given two events A and B, the probability that either A or B occurs is given by:
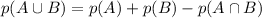
where
 the probability that A occurs
the probability that A occurs
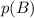 is the probability that B occurs
is the probability that B occurs
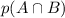 is the probability that both A and B occur at the same time
is the probability that both A and B occur at the same time
In this problem, we know the following facts:
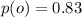 is the probability that the car requires an oil change
is the probability that the car requires an oil change
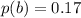 is the probability that the car requires a brake repair
is the probability that the car requires a brake repair
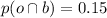 is the probability that the car requires both an oil change and brake repair
is the probability that the car requires both an oil change and brake repair
Therefore, the probability that either o (car requiring oil change) or b (car requiring brake repait) occur is: