Answer:
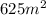
Explanation:
In this problem, we are told that the area of the garden is given by the expression
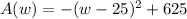
where
w is the width of the garden (in meters)
Here we want to find the maximum possible area.
The maximum of a function f(x) can be found by requiring that its first derivative is zero:
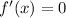
Therefore, here we have to calculate the derivative of
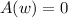 and find the value of w for which it is equal to zero.
and find the value of w for which it is equal to zero.
Let's start by rewriting the area function as

Now we calculate the derivative with respect to w:

Now we require this derivative to be zero, so
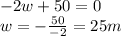
So now we can substitute this value of w into the expression of A(w) to find the maximum possible area:
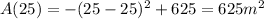
This value is allowed because we know that the maximum length of the perimeter of the fence is 100 meters; If the garden has a square shape, the length of each side is
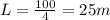 , and the area of the squared garden is
, and the area of the squared garden is
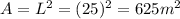
Which is equal to what we found earlier: this means that the maximum area is achieved if the garden has a squared shape.