Number of 25 cents coins is 80 and number of 50 cents coins is 20.
Explanation:
Given,
A bag contains of total value $ 30.
Number of 25 cent coins is four times that of 50 cent coins.
To find number of 25 cents coin is x
Number of 50 cents coin is y
According to the problem,
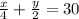 ----- eq 1
----- eq 1
x = 4y------ eq 2
Now, we will solve these two equation.
Putting the values of x = 4y in eq 1 we get,
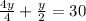
or,
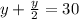
or,
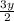 = 30
= 30
or, y = 20
So, x = 4×20 = 80
Hence,
Number of 25 cents coins = 80 and number of 50 cents coins = 20