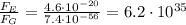Answer:
The electric force is
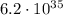 times stronger than the gravitational force
times stronger than the gravitational force
Step-by-step explanation:
The magnitude of the electrostatic force between two charges is given by:
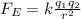
where:
 is the Coulomb's constant
is the Coulomb's constant
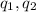 are the two charges
are the two charges
r is the separation between the two charges
In this problem:
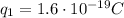 (charge of the proton)
(charge of the proton)
 (charge of a nucleus of helium, twice the charge of a proton)
(charge of a nucleus of helium, twice the charge of a proton)
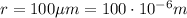
So the electric force is
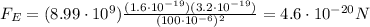
Instead, the magnitude of the gravitational force between two objects is given by :
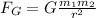
where
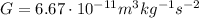 is the gravitational constant
is the gravitational constant
m1, m2 are the masses of the two objects
r is the separation between them
Here we have:
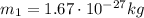 is the mass of the proton
is the mass of the proton
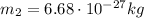 is the mass of a nucleus of helium (4 times the mass of the proton)
is the mass of a nucleus of helium (4 times the mass of the proton)
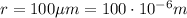 is the separation
is the separation
So the gravitational force is
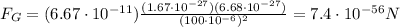
So, we see that the electric force is much stronger than the gravitational factor, by a factor of: