Question

Given the following use a Pythagorean identity to find tan(0) if 0 is in quadrant II

Answer 1

tan θ = -√7/3

Explanation:

We have the equation as following:

`sin^(2) x + cos^(2) x =1`

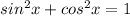
=> (cos x)^ 2 = 1 - (sin x)^2

So that:

(cos θ)^2 = 1 - (sin θ)^2 = 1- (√7/4)^2 = 1 - 7/16 = 9/16

=> (cos θ)^2 = 9/16

We have the equation:

`tan^(2) x = (1)/(cos^(2)x ) -1`

=> (tan θ)^2 = 1/(cos θ)^ 2 - 1 = 1/(9/16 ) - 1 = 16/9 - 1 = 7/9

=> tan θ = √7/3 or tan θ = -√7/3

As θ is in the quadrant II, so that tan θ would have the negative value

=> tan θ = -√7/3

Answer C is correct