Explanation:
A quadratic function is a second-degree polynomial function with the general form
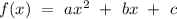 ,
,
where
 ,
,
 , and
, and
 are real numbers, and
are real numbers, and
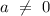 .
.
The standard form or the vertex form of a quadratic function is, however, a little different from the general form. To get the standard form from the general form, we need to use the "complete the square" method.
![f(x) \ = \ ax^(2) \ + \ bx \ + \ c \\ \\ \\ f(x) \ = \ a\left(x^(2) \ + \ \displaystyle(b)/(a)x \right) \ + \ c \\ \\ \\ f(x) \ = \ a\left[x^(2) \ + \ \displaystyle(b)/(a)x \ + \ \left(\displaystyle(b)/(2a)\right)^(2) \ - \ \left(\displaystyle(b)/(2a)\right)^(2) \right] \ + \ c \\ \\ \\ f(x) \ = \ a\left[x^(2) \ + \ \displaystyle(b)/(a)x \ + \ \left(\displaystyle(b)/(2a)\right)^(2)\right] \ - \ a\left(\displaystyle(b)/(2a)\right)^(2) \ + \ c](https://img.qammunity.org/2023/formulas/mathematics/college/hsi6afgmjzh3dcj4mtnf7ntryqx6j1kldn.png)
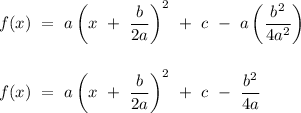
Let
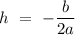 and
and
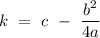 ,
,
then the expression reduces into
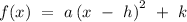 ,
,
where the point (h, k) are the coordinates for the vertex of the quadratic function.
There are two different methods to approach this question. First, we consider the general form of the quadratic function, it is observed that has a y-intercept at the point
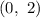 , so
, so
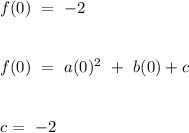 .
.
Additionally, it is pointed that two distinct points
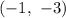 and
and
 lies on the quadratic graph, hence
lies on the quadratic graph, hence
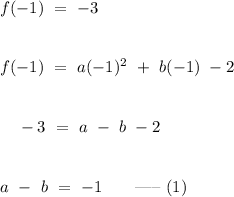
and
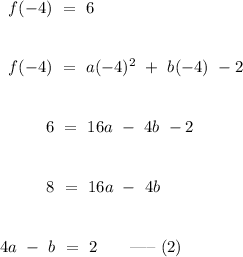 .
.
Subtract equation (1) from equation (2) term-by-term,
![\-\hspace{0.72cm} (4a \ - \ b) \ - \ (a \ - \ b) \ = \ 2 \ - \ (-1) \\ \\ \\ (4a \ - \ a) \ + \ \left[-b \ - \ (-b)\right] \ = \ 2 \ + \ 1 \\ \\ \\ \-\hspace{3.8cm} 3a \ = \ 3 \\ \\ \\ \-\hspace{4cm} a \ = \ 1](https://img.qammunity.org/2023/formulas/mathematics/college/fdklkkv2yrrcimtiesrvguatnqwzp0ad13.png)
Substitute
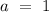 into equation (1),
into equation (1),
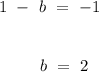 .
.
Therefore, the equation of the quadratic function is
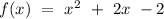 .
.

Alternatively, the vertex of the quadratic function is given as the point
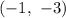 , substitute these coordinates into the vertex form of a quadratic function.
, substitute these coordinates into the vertex form of a quadratic function.
 .
.
Substitute the point
 into the function above,
into the function above,
![f(-4) \ = \ 6 \\ \\ \\ f(-4) \ = \ a\left[(-4) \ + \ 1\right]^(2) \ - \ 3 \\ \\ \\ \-\hspace{0.75cm} 6 \ = \ a(-3)^(2) \ - \ 3 \\ \\ \\ \-\hspace{0.55cm} 9a \ = \ 9 \\ \\ \\ \-\hspace{0.75cm} a \ = \ 1](https://img.qammunity.org/2023/formulas/mathematics/college/a0seyj41j33oegfkjbr8zmmio0fr9laxrm.png) .
.
Therefore, the general form of the quadratic function is
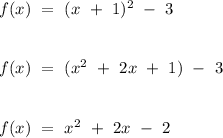 .
.