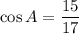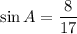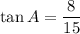Without the figure we can't tell which angle A is. We'll write it both ways; you'll have to match the appropriate one to your problem.
If A is the angle opposite 17 then its the right angle. They're probably not giving us that problem.
If A is the angle opposite 15 then


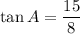
If A's the one opposite 7 then cosine and sine swap and tangent gets flipped: