Question

This is due today, and I have no idea what I'm doing, please help if you know anything.

Answer 1

Binomial distribution formula: P(x) = (n k) p^k * (1 - p)^n - k

a) Probability that four parts are defective = 0.01374

P(4 defective) = (25 4) (0.04)^4 * (0.96)^21

P(4 defective) = 0.01374

b) Probability that at least one part is defective = 0.6396

Find the probability that 0 parts are defective and subtract that probability from 1.

P(0 defective) = (25 0) (0.04)^0 * (0.96)^25

P(0 defective) = 0.3604

1 - 0.3604 = 0.6396

c) Probability that 25 parts are defective = approximately 0

P(25 defective) = (25 25) (0.04)^25 * (0.96)^0

P(25 defective) = approximately 0

d) Probability that at most 1 part is defective = 0.7358

Find the probability that 0 and 1 parts are defective and add them together.

P(0 defective) = 0.3604 (from above)

P(1 defective) = (25 1) (0.04)^1 * (0.96)^24

P(1 defective) = 0.3754

P(at most 1 defective) = 0.3604 + 0.3754 = 0.7358

e) Mean = 1 | Standard Deviation = 0.9798

mean = n * p

mean = 25 * 0.04 = 1

stdev =
`√(np(1-p))`

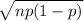
stdev =
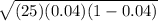`√((25)(0.04)(1-0.04))` = 0.9798

Hope this helps!! :)