Given that the function is

We need to determine the average rate of change over the interval
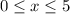
Value of f(x) when x = 0:
Substituting x = 0 in the function
 , we have;
, we have;

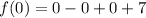
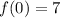
Thus, the value of f(0) is 7.
Value of f(x) when x = 5:
Substituting x = 5 in the function
 , we have;
, we have;
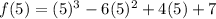
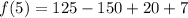
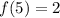
Thus, the value of f(5) is 2.
Average rate of change:
The average rate of change can be determined using the formula,

where
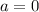 and
and
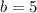
Thus, we have;
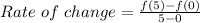

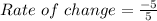
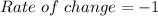
Thus, the average rate of change over the interval
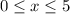 is -1.
is -1.