Answer:
99% confidence interval for the given specimen is [3.4125 , 3.4155].
Explanation:
We are given that a laboratory scale is known to have a standard deviation (sigma) or 0.001 g in repeated weighing. Scale readings in repeated weighing are Normally distributed with mean equal to the true weight of the specimen.
Three weighing of a specimen on this scale give 3.412, 3.416, and 3.414 g.
Firstly, the pivotal quantity for 99% confidence interval for the true mean specimen is given by;
P.Q. =
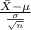 ~ N(0,1)
~ N(0,1)
where,
 = sample mean weighing of specimen =
= sample mean weighing of specimen =
 = 3.414 g
= 3.414 g
 = population standard deviation = 0.001 g
= population standard deviation = 0.001 g
n = sample of specimen = 3
 = population mean
= population mean
Here for constructing 99% confidence interval we have used z statistics because we know about population standard deviation (sigma).
So, 99% confidence interval for the population mean,
 is ;
is ;
P(-2.5758 < N(0,1) < 2.5758) = 0.99 {As the critical value of z at 0.5% level
of significance are -2.5758 & 2.5758}
P(-2.5758 <
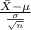 < 2.5758) = 0.99
< 2.5758) = 0.99
P(
 <
<
 <
<
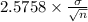 ) = 0.99
) = 0.99
P(
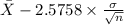 <
<
 <
<
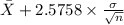 ) = 0.99
) = 0.99
99% confidence interval for
 = [
= [
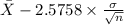 ,
,
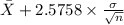 ]
]
= [
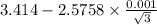 ,
,
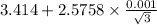 ]
]
= [3.4125 , 3.4155]
Therefore, 99% confidence interval for this specimen is [3.4125 , 3.4155].