Question

A straight trail with a uniform inclination of 15 degrees leads from a lodge at an elevation of 600 feet to a mountain lake at an elevation of 6100 feet. What is the length of the trail (to the nearest foot)

Answer 1

The length of the trail = 22796 ft

Step-by-step explanation:

From the ΔABC

AC = length of the trail = x

AB = 6100 - 600 = 5500 ft

Angle of inclination
`\theta` = 15°

`\sin \theta = (AB)/(AC)`

`\sin 15 = (5900)/(x)`

`x = (5900)/(0.2588)`

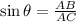
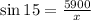
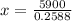
x = 22796 ft

Since x = AC = Length of the trail.

Therefore the length of the trail = 22796 ft