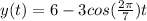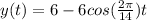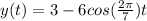Question

The Hudson Bay tides vary between 3 feet and 9 feet. The tide is at its lowest point when time (t) is 0 and completes a full cycle in 14 hours. What is the amplitude, period, and midline of a function that would model this periodic phenomenon? Amplitude = 3 feet; period = 14 hours; midline: y = 6 Amplitude = 3 feet; period = 7 hours; midline: y = 3 Amplitude = 6 feet; period = 14 hours; midline: y = 6 Amplitude = 6 feet; period = 7 hours; midline: y = 3

Answer 1

`y(t) = 6 -3cos((2\pi )/(14) )t`

`y(t) = 6 -3cos((2\pi )/(7) )t`

`y(t) = 6 - 6cos((2\pi )/(14) ) t`

`y(t) = 3- 6cos((2\pi )/(7) )t`

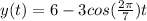
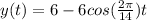
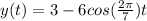
Explanation:

Given that,

Hudson Bay tides vary between
`3 ft` and
`9 ft`.

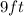Tide is at its lowest when
`t=0`

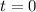
Completes a full cycle in 14 hours.

To find:- What is the amplitude, period, and midline of a function that would model this periodic phenomenon?

So, The periodic function of this model is

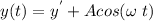`y(t) = y^(') + Acos(\omega\ t)` ...................(1)

where,
`A- Amplitude of cycle`

`\omega = Angular speed (in Radian.)`

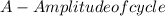
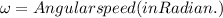
Then putting the value in given Equation(1) we get,

Amplitude =
`(9-3)/(2) ft = 3ft`

`y^(') = (3+ 3 )ft = 6ft`

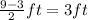
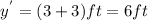
Now, At
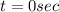`t=0 sec` it complete full cycle in
`14 hours.`
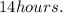
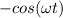`-cos(\omega t)` because it is at lowest at t=0sec.

∵
`\omega t= 2\pi`

`\omega (t+14) = 2\pi`

∴
`\omega = (2\pi )/(14)`

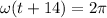
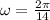
Hence
`y(t) = 6 -3cos((2\pi )/(14) )t`

`y(t) = 6 -3cos((2\pi )/(7) )t`

`y(t) = 6 - 6cos((2\pi )/(14) ) t`

`y(t) = 3- 6cos((2\pi )/(7) )t`