Answer:
The p-value is
- Select your answer -
between .025 and .05
between .05 and .025
between .015 and .045
What is your conclusion?
- Select your answer -
Conclude church attendance is not independent of age.
Conclude church attendance is independent of age.
What conclusion can you draw about church attendance, as individuals grow older?
- Select your answer -
Church attendance increases as individuals grow older.
Church attendance decreases the grow older.
Explanation:
Hello!
There was a survey conducted where the people were asked two questions if they attended church and their age, both answers were recorded, so there are two variables of interest:
X₁: Church attendance, categorized: "Yes" and "No"
X₂: Age of the surveyed person, categorized: "20 to 29", "30 to 39", "40 to 49" and "50 to 59"
The objective is to test if both variables are independent so the hypothesis is:
H₀: Pij= Pi. * P.j ∀ i= 1, 2 and j= 1, 2, 3, 4
H₁: The categorized variables are not independent.
α: 0.05
Tho test this you have to conduct a Chi-Square test of independence, and the statistic is:
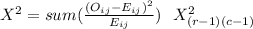
Before calculating the value of the statistic under the null hypothesis you have to calculate all expected frequencies using the following formula:
Eij= (Oi.*O.j)/n
E₁₁= (O₁.*O.₁)/n= (260*100)/600= 43.33
E₁₂= (O₁.*O.₂)/n= (260*150)/600= 65
E₁₃= (O₁.*O.₃)/n= (260*200)/600= 86.67
E₁₄= (O₁.*O.₄)/n= (260*150)/600= 65
E₂₁= (O₂.*O.₁)/n= (340*100)/600= 56.67
E₂₂= (O₂.*O.₂)/n= (340*150)/600= 85
E₂₃= (O₂.*O.₃)/n= (340*200)/600= 113.33
E₂₄= (O₂.*O.₄)/n= (340*150)/600= 85
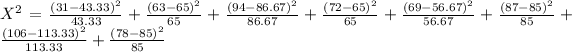
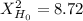
The rejection region for this test is one-tailed to the right with critical value:
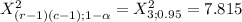
The p-value for this test is also one-tailed and is
p-value: 0.033255
This p-value is less than the significance level, so the decision is to reject the null hypothesis. At a level of 5%, you can say that church attendance and the age of the people surveyed are not independent.
I hope it helps!