Question

Jasmyn is standing at the point of tangency to a wishing

well, 27 feet from a bench. Her friend Willard is at the other
point of tangency. Determine the value of x.
w
(2x - 7) ft
27 ft
Sac
​

Answer 1

The value of
`x=17 ft`.

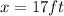Explanation:

Diagram of the given scenario shown below.

Given that,

Distance from bench to Jasmyn is
`PA=27ft`.

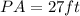Distance from bench to Willard is
`PB=(2x-7)ft`.

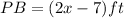From the Question,

The given wishing well is a circle and all the distance are tangent to the circle.

So, Triangle formed by these points are such as Δ
`PAO` and Δ
`PBO`.

Now, taking both Δ
`PAO` and Δ
`PBO`.

⇒
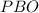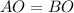`AO = BO` {Radius of circle are equal}

⇒
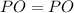`PO=PO` {Common side}

⇒ ∠
`PAO =` ∠
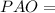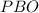`PBO` {radius is always perpendicular

to the point of tangent }

∴ Δ
`PAO` ≅ Δ
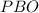`PBO` {By SAS congruence theorem}

Therefore,
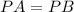`PA=PB` ....(1) {corresponding part of

congruence triangle (CPCT)}

Thus,

⇒
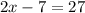`2x-7=27` {from equation (1)}

⇒
`2x=34`

⇒
`x=17 ft`

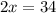
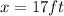
Hence, The value of
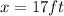`x=17 ft`.