The question is incomplete, here is the complete question:
Calculate the pH at 25°C of a 0.39 M solution of sodium hypochlorite NaClO. Note that hypochlorous acid HClO is a weak acid with a pKa of 7.50. Round your answer to 1 decimal place.
Answer: The pH of the solution is 10.4
Step-by-step explanation:
We are given:
Molarity of sodium hypochlorite = 0.39 M
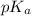 of HClO = 7.50
of HClO = 7.50
We know that:
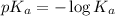
 of HClO =
of HClO =
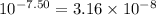
To calculate the base dissociation constant for the given acid dissociation constant, we use the equation:
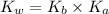
where,
 = Ionic product of water =
= Ionic product of water =
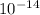
 = Acid dissociation constant =
= Acid dissociation constant =
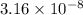
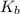 = Base dissociation constant
= Base dissociation constant
Putting values in above equation, we get:
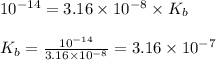
The chemical equation for the reaction of hypochlorite ion with water follows:

Initial: 0.39
At eqllm: 0.39-x x x
The expression of
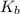 for above equation follows:
for above equation follows:
![K_b=([HClO][OH^-])/([ClO^-])](https://img.qammunity.org/2021/formulas/chemistry/high-school/lfsokwtsk97qkpxnebz493h9egpgd2wfgw.png)
Putting values in above equation, we get:
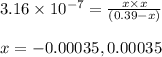
Neglecting the negative value of 'x' because concentration cannot be negative
To calculate the pOH of the solution, we use the equation:
![pOH=-\log[OH^-]](https://img.qammunity.org/2021/formulas/chemistry/high-school/aptpm2b2equoweomw80psbpn50765hcb2n.png)
We are given:
![[OH^-]=0.00035M](https://img.qammunity.org/2021/formulas/chemistry/high-school/di02u5cw0bi0jfh3erdolnve3q7rpolrdi.png)
Putting values in above equation, we get:
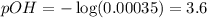
To calculate pH of the solution, we use the equation:
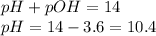
Hence, the pH of the solution is 10.4