Question

The areas of the squares adjacent to two sides of a right triangle are 29.2529.2529, point, 25 units^2 2 squared and 131313 units^2 2 squared. Find the length, xxx, of the third side of the triangle.

Answer 1

It is 6.5 units

Answer 2

6.5 units

Explanation:

We are given that

Two adjacent sides of right triangle

Suppose a,b and c are sides of right triangle.

`a^2=29.25`squared units

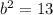`b^2=13`square units.

Length of third side,c=x units

We have to find the length of third side of the triangle.

`x^2=a^2+b^2`

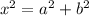
By using Pythagoras theorem

`(Hypotenuse)^2=(Perpendicular\;side)^2+(Base)^2`

`x^2=29.25+13`

`x=√(29.25+13)`

`x=6.5units`

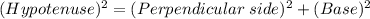
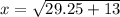
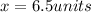
Hence, the length x of the third side of the triangle=6.5 units