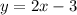Linear Equations
Linear equations are typically organized in slope-intercept form:

- m = the slope
- b = the y-intercept (the value of y when x=0)
To find the slope of a line (m):
- Find the coordinates of two points that fall on the line
- Plug them into the following formula:
 where
where
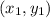 and
and
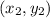 are the coordinates of the points
are the coordinates of the points
To find the y-intercept of a line (b):
- Plug the slope into

- Plug in one of the points
- Solve for b
Solving the Question
We're given a set of coordinates for this linear relation.
First, solve for the slope.

⇒ Plug in any two points that fall on the line (in this case we used (-2,-7) and (2,1)):
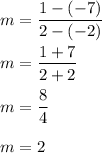
Therefore, the slope of the line is 2. Plug this into
 :
:

Now, let's solve for the y-intercept (b)

Plug in any of the given points:
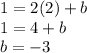
Therefore, the y-intercept of the line is 3. Plug this back into
 :
:
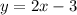
Answer