Question

A toroid having a square cross section, 5.49 cm on a side, and an inner radius of 18.1 cm has 450 turns and carries a current of 0.859 A. (It is made up of a square solenoid instead of round one bent into a doughnut shape.) What is the magnitude of the magnetic field inside the toroid at (a) the inner radius and (b) the outer radius

Answer 1

(a) 4.27 x 10^-4 Telsa

(b) 3.28 x 10^-4 Telsa

Step-by-step explanation:

side of square, a = 5.49 cm

inner radius, r = 18.1 cm = 0.181 m

number of turns,N = 450

current, i = 0.859 A

(a)

The magnetic field due to a solenoid due to inner radius is

`B = (\mu_(0)Ni)/(2\pi r_(inner))`

`B = (4\pi* 10^(-7)* 450* 0.859)/(2\pi * 0.181)`

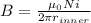
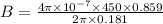
B = 4.27 x 10^-4 Telsa

(b)

The outer radius is R = 18.1 + 5.49 = 23.59 cm = 0.236 m

The magnetic field due to the outer radius is

`B = (\mu_(0)Ni)/(2\pi r_(outer))`

`B = (4\pi* 10^(-7)* 450* 0.859)/(2\pi * 0.236)`

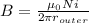
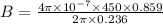
B = 3.28 x 10^-4 Tesla